The effects of feedback in systems on other working parameters of the system has been analysed. The parameter considered for analysis are gain, sensitivity, distortion, impedance and bandwidth. A feedback system has been shown in the figure.

Effect of feedback on overall Gain:

From figure it is seen that the transfer function is given by the equation:

M= A/(1- bA)

Hence the feedback reduces the overall gain of the system by a factor of (1-bA).

The quantities A and B are function of frequency and can be adjusted to make the denominator greater than unity.

Hence the gain increases for a particular frequency range and decreases for another frequency range.

Effect of Feedback on Sensitivity:

Sensitivity is the extent to which the system responds to changes in parameters like gain,impedance,etc. Sensitivity is also said to be the ratio of the extent of change of one of the above mentioned parameter to a small change of the determining parameters.

For example, if

M= transfer function

K= Determining Parameter

Then the sensitivity (S) is given by:

S= Percentage change in M/ Percentage change in K

Following are the effect of feedback on Sensitivity.

Feedback may reduce sensitivity with respect to certain parameters.

Feedback does not affect variations of elements in the feedback path.

Feedback reduces the sensitivity of the system based on variation of parameter in the forward path of the loop. Larger the loop gain Ab, more effective is the feedback in reducing sensitivity.

Effect of Feedback on Distortion

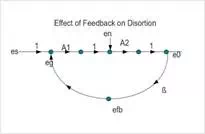
Feedback is used in communication systems to reduce noise and other distortion signals which it might pickup from extraneous sources.

The place of insertion of the extraneous noise to the signal flow is the main factor that determines the extent to which the feedback reduces the effects due to distortion.

Consider the figure which shows a signal flow graph of a system.

A noise signal is inserted at the point shown.

In the absence of the feedback, the output is given by

e0 = A1A2es + A2en

= e0s + e0n

where, e0s = single component of the output.

e0n=The component of the output due to noise.

Output signal to noise ration = output due to signal/output due to noise

Signal to noise ratio = A1A2es/A2en

= A1es/en

Hence to increase the signal to noise ratio, either A1 and /or es is to be increased or en is to be decreased.

If the system is aided by a feedback circuit, the output is given by:

e0 = (A1A2es/1-A1A2b) + (A2en/1-A1A2b)

From the above equation it is clear that the noise component of the output has its gain reduced by a factor 1-A1A2b. Thus the noise is reduced and the overall distortion of the output is reduced.

Effect of Feedback on Impedance

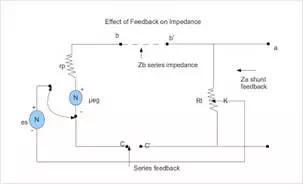
In practice , the system is bound be connected to an external circuit. The working of the system depends on the input and output impedance. Consider the figure shown.

Za = (rpRl)/(rp+Rl+µKRl)

Here the amplifier gain is given by:

A = – µRl/(rp + Rl)

Thus the shunt impedance is reduced by a factor (1-AK). The series impedance is increased by a factor (1- AK).

Effect of Feedback on Bandwidth

In electronic frequency dependent circuits, bandwidth is an important characteristic.

Bandwidth is the parameter that measure the ability of the system to reproduce its input signal with high quality and least noise.

It is to be noted that the bandwidth increases with feedback.